SURF159 Element Description

Use SURF159 to model axisymmetric solid surface loads acting on general axisymmetric solid elements (SOLID272 or SOLID273). The element has linear or quadratic displacement behavior on the master plane and is well suited to modeling irregular meshes on the master plane. It is defined by two or three nodes on the master plane, and nodes created automatically in the circumferential direction based on the master plane nodes. The total number of nodes depends on the number of nodal planes (KEYOPT(2)). The element area between nodal planes is called a facet. Each node has three degrees of freedom: translations in the nodal x, y and z directions. Various loads and surface effects can exist simultaneously.

In addition to the SURF159 element information provided here, the following topics are available in the Mechanical APDL Theory Reference:

For related information (concerning the elements used with SURF159), see General Axisymmetric Elements in this document.

Figure 159.1: SURF159 Geometry (KEYOPT(2) = 3)

SURF159 Input Data

The geometry and node locations for this element (when KEYOPT(2) = 3) are shown in Figure 159.1: SURF159 Geometry (KEYOPT(2) = 3). The element input data includes nodes, real constants, and the material properties. The total number of nodes is the two or three base nodes times the number of nodal planes. (For information about how Fourier nodes are generated, see the NAXIS command documentation.) SURF159 elements can be generated via the ESURF command before generating nodes for general axisymmetric element sections (NAXIS).

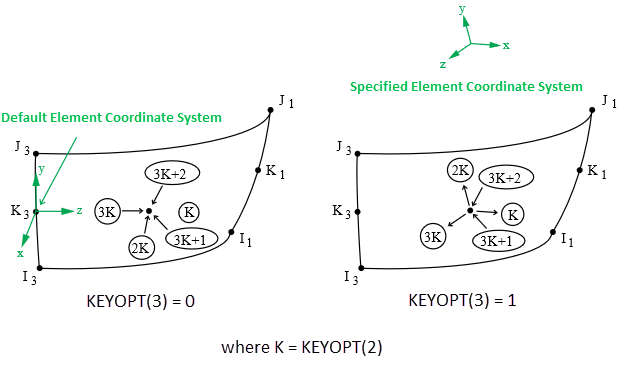
The element has the x-axis as normal to the element surface, the y-axis in the meridional direction, and the z-axis in the circumferential direction.

The mass and volume calculations use the element thicknesses (real constants TKI, and TKJ). Thickness TKJ defaults to TKI. The mass calculation uses the density (material property DENS, mass per unit volume) and the real constant ADMSUA, the added mass per unit area.

The stress stiffness matrix and load vector calculations use the in-plane force per unit length (input as real constant SURT) and the elastic foundation stiffness (input as real constant EFS); the EFS uses pressure-per-length (or force-per-length-cubed) units. The foundation stiffness can be damped, either by using the material property BETD as a multiplier on the stiffness or by directly using the material property VISC.

"SURF159 Input Summary" contains a summary of the element input. See Element Input for a general description of element input.

Pressure Loads

Pressures must be input as element surface loads (as force-per-length squared) on the element faces as shown by the circled numbers in Figure 159.2: SURF159 Pressures. For SURF159, applying surface loads to the element (SFE) or nodes (SF) is very different in comparison to the SOLID272 and SOLID273 elements. An SF command, if issued, applies loads to faces 1 through K only (where K = KEYOPT(2), the number of nodal planes).

SURF159 allows complex loads.

Defaults for Pressures on Faces 1 through 3K

If the first pressure for a face is specified (SFE) and the other three remain blank, the other three pressures default to the first.

If values are specified (and perhaps supplemented by the aforementioned default) to the first face of each of the three face families (1 through K, K+1 through 2K, or 2K+1 to 3K), the same values are assigned to all other faces around the circumference. (The SFELIST command does not reflect this defaulting behavior.)

Example 1  —  To obtain a uniform pressure across the element and around the circumference, only one specified pressure is necessary on the first face of the face family.

Example 2  —  To obtain a pressure that tapers in the radial direction and is constant around the circumference, all four values must be specified on the first face of the face family.

Faces 1 through 3K [KEYOPT(3) = 0]  —  Positive values of pressure on these faces act in the positive element coordinate directions, as follows: faces 1 through K in the element x (normal) direction, faces K+1 through 2K in the element y (tangent, meridional) direction, and faces 2K+1 through 3K in the element z (tangent, circumferential) direction. For faces 1 through K, positive or negative values may be removed as requested via KEYOPT(6) to simulate the discontinuity at the free surface of a contained fluid. For faces K+1 through 3K, the direction of the load is controlled by the element coordinate system; therefore, the ESYS command may be needed.

Faces 1 through 3K [KEYOPT(3) = 1]  —  Pressure loads are applied to the element faces according to the local coordinate system, as follows: faces 1 through K in the local x direction, faces K+1 through 2K in the local y direction, and faces 2K+1 through 3K in the local z direction. A local coordinate system must be defined, and the element must be set to that coordinate system via the ESYS command. KEYOPT(6) does not apply.

Figure 159.2: SURF159 Pressures

Face 3K+1  —  The direction is normal to the element and the magnitude of the pressure at each integration point is P_I + XP_J + YP_K + ZP_L, where P_I through P_L are input as VAL1 through VAL4 on the SFE command, and X, Y, Z are the global Cartesian coordinates at the current location of the integration point. No input values can be blank. Positive or negative values may be removed as requested with KEYOPT(6) to simulate the discontinuity at the free surface of a contained fluid. The SFFUN and SFGRAD commands do not work with face 3K+1.

Face 3K+2  —  The magnitude of the pressure is P_I, and the direction is where i, j, and k are unit vectors in the global Cartesian directions. The load magnitude may be adjusted with KEYOPT(11) and KEYOPT(12). No input values can be blank.

Temperatures may be input as element body loads at the nodes. For the nodes on the master plane, the node I₁ temperature T(I₁) defaults to TUNIF. If all other temperatures are unspecified, they default to T(I₁). If both corner node temperatures are specified, the midside node temperature defaults to the average temperature of the adjacent corner nodes. For any other input pattern, unspecified temperatures default to TUNIF. For the nodes generated in the circumferential direction based on the master node, if all other temperatures are unspecified, they default to the value of their base nodes (T(I₁), T(J₁), and T(K₁), depending on their location). For any other input pattern, unspecified temperatures default to TUNIF.

The effects of pressure load stiffness are automatically included for this element. If an unsymmetric matrix is needed for pressure load stiffness effects, issue a NROPT,UNSYM command. For a geometric nonlinear analysis when convergence is an issue, use an unsymmetric matrix.

SURF159 Output Data

The solution output associated with the element is in two forms:

A general description of solution output is given in Solution Output. See the Basic Analysis Guide for ways to view results.

To view 3D mode shapes for a modal or eigenvalue buckling analysis, expand the modes with element results calculation active (via the MXPAND command's Elcalc = YES option).

The Element Output Definitions table uses the following notation:

A colon (:) in the Name column indicates that the item can be accessed by the Component Name method (ETABLE, ESOL). The O column indicates the availability of the items in the file jobname.out. The R column indicates the availability of the items in the results file.

In either the O or R columns, “Y” indicates that the item is always available, a letter or number refers to a table footnote that describes when the item is conditionally available, and “-” indicates that the item is not available.

Table 159.3: SURF159 Item and Sequence Numbers lists output available through ETABLE using the Sequence Number method. See Creating an Element Table in the Basic Analysis Guide and The Item and Sequence Number Table in this document for more information. The following notation is used in the output table:

SURF159 Assumptions and Restrictions

SURF159 Product Restrictions

There are no product-specific restrictions for this element.