VM308
VM308
Steady-State Heat Conduction in Functionally Graded Hollow Cylinder and Hollow Sphere
Overview
| Reference: | Yoshihiro Obata and Naotake Noda. "Steady Thermal Stresses in a Hollow Circular Cylinder and a Hollow Sphere of a Functionally Gradient Material." Journal of Thermal Stresses. 1994: 17:3, 471-487. |
| Analysis Type(s): | Steady-State Thermal (ANTYPE = 0) |
| Element Type(s): |
2D 4-Node Thermal Solid Element (PLANE292) 2D 6-Node Thermal Solid Element (PLANE293) |
| Input Listing: | vm308.dat VM308 requires a supplemental .cdb input file which is too long to include full input listings. This file must be downloaded and placed in your working directory for the test case to run properly. Additionally, the geometry and mesh should be regenerated. Download link: MAPDL Test Case Files for 2024 R2 vm308.cdb |
Test Case
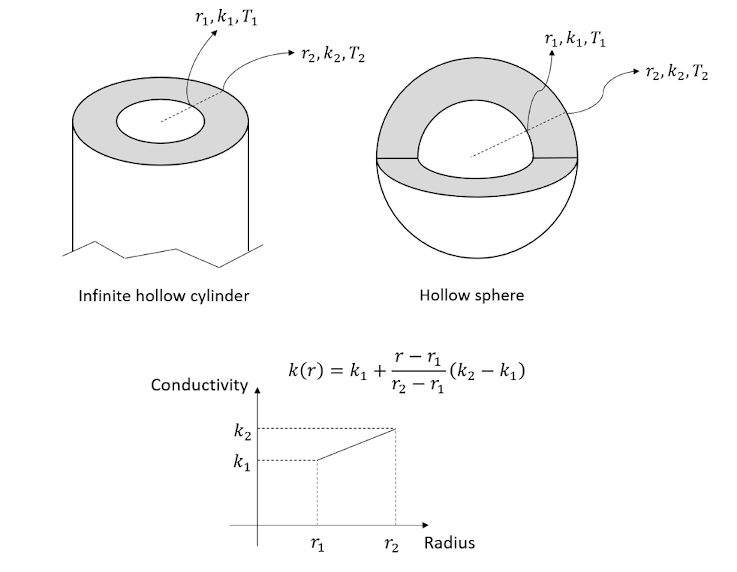
An infinite hollow cylinder has an inside radius r1, outside radius r2, and temperature T1 on the inside surface, and temperature T2 on the outside surface. The cylinder has a functionally graded material (FGM) exhibiting variable thermal conductivity. The conductivity on the inner surface is denoted by k1 and on the outer surface by k2. The mixture composition of FGM varies along the thickness in such a way that thermal conductivity is a linear function of radius.
Similarly, a hollow sphere with prescribed temperatures on the inside and outside surfaces is considered. It is made of an FGM material and has linearly varying thermal conductivity along the radius.
Determine the temperature variation along the radii of the hollow infinite cylinder and the hollow sphere.
Analysis Assumptions and Modeling Notes
The hollow cylinder and sphere are modeled as 2D domains with axisymmetric option. The hollow cylinder is modeled with the PLANE292 2D 4-node thermal solid element. The hollow sphere is modeled with the PLANE293 2D 6-node thermal solid element (KEYOPT(1) = 1).
The radius dependency of conductivity is realized by defining field variables for material data tables, a capability available only with current-technology elements. For the hollow cylinder, the field variable is defined to be the radius (TBFIELD,XCOR). For the hollow sphere, a user-defined field variable is specified ((TBFIELD,UF09), where UF09 is defined as node-based initial-state data (INISTATE)) and is set equal to the radius.
A steady-state (static) thermal analysis determines the temperature variation along the radius, which is then compared with the analytical solution.
Results Comparison
Hollow Cylinder
| Radius (m) | Target (ᵒC) | Mechanical APDL (ᵒC) | Ratio |
|---|---|---|---|
| 0.100 | 100.000 | 100.000 | 1.0000 |
| 0.102 | 95.092 | 95.048 | 0.9995 |
| 0.104 | 89.894 | 89.811 | 0.9991 |
| 0.106 | 84.351 | 84.243 | 0.9987 |
| 0.108 | 78.393 | 78.288 | 0.9987 |
| 0.110 | 71.928 | 71.881 | 0.9993 |
| 0.112 | 64.831 | 64.682 | 0.9977 |
| 0.114 | 56.927 | 56.677 | 0.9956 |
| 0.116 | 47.962 | 47.629 | 0.9931 |
| 0.118 | 37.540 | 37.211 | 0.9912 |
| 0.120 | 25.000 | 25.000 | 1.0000 |
Hollow Sphere
| Radius (m) | Target (ᵒC) | Mechanical APDL (ᵒC) | Ratio |
|---|---|---|---|
| 0.100 | 100.000 | 100.000 | 1.0000 |
| 0.102 | 94.585 | 94.530 | 0.9994 |
| 0.104 | 88.962 | 88.871 | 0.9990 |
| 0.106 | 83.080 | 83.030 | 0.9994 |
| 0.108 | 76.876 | 76.786 | 0.9988 |
| 0.110 | 70.267 | 70.102 | 0.9977 |
| 0.112 | 63.143 | 63.003 | 0.9978 |
| 0.114 | 55.350 | 55.261 | 0.9984 |
| 0.116 | 46.664 | 46.401 | 0.9944 |
| 0.118 | 36.740 | 36.446 | 0.9920 |
| 0.120 | 25.000 | 25.000 | 1.0000 |