VM254
VM254
Campbell Diagrams and Critical Speeds Using Symmetric
Orthotropic Bearings
Test Case
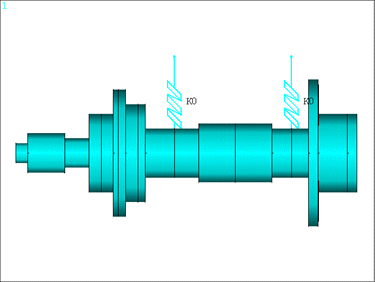
A rotor-bearing system is analyzed to determine the forward
and backward whirl speeds. The distributed rotor was modeled as a
configuration of six elements with each element composed of subelements.
See Table 11: Geometric Data of Rotor-Bearing Elements for a list of the geometrical data
of the elements. Two symmetric orthotropic bearings were located
at positions four and six. Modal analysis is performed on rotor bearing
system with multiple load steps to determine the whirl speeds and
Campbell values for the system.
Table 11: Geometric Data of Rotor-Bearing Elements
| Element No. | Subelement No. | Axial Distance to Subelement (cm) | Inner Diameter (cm) | Outer Diameter (cm) |
|---|
| 1 | 1 | 0.00 | | 1.02 |
| 2 | 1.27 | | 2.04 |
| 2 | 1 | 5.08 | | 1.52 |
| 2 | 7.62 | | 4.06 |
| 3 | 1 | 8.89 | | 4.06 |
| 2 | 10.16 | | 6.60 |
| 3 | 10.67 | 1.52 | 6.60 |
| 4 | 11.43 | 1.78 | 5.08 |
| 5 | 12.70 | | 5.08 |
| 6 | 13.46 | | 2.54 |
| 4 | 1 | 16.51 | | 2.54 |
| 2 | 19.05 | | 3.04 |
| 5 | 1 | 22.86 | | 3.04 |
| 2 | 26.67 | | 2.54 |
| 6 | 1 | 28.70 | | 2.54 |
| 2 | 30.48 | | 7.62 |
| 3 | 31.50 | | 4.06 |
| 4 | 34.54 | 1.52 | 4.06 |
Analysis Assumptions and Modeling Notes
A modal analysis is performed on a rotor bearing system with
DAMP (PIPE16) and QR Damp (PIPE288) methods to determine the whirl speeds and Campbell values. PIPE16 and PIPE288 elements
are used to model the rotor shaft, MASS21 elements are used to model the rigid disk (concentrated mass), and COMBI214 elements are used to model symmetric bearings.
No shear effect is included in the rotor-bearing system. The displacement
along X as well as the rotation around X axis is constrained so that
the rotor bearing system does not have any torsion or traction related
displacements. The CORIOLIS command is activated
in a stationary reference frame to apply gyroscopic effect to the
rotating structure. The backward and forward whirl speeds are determined
from modal analysis and compared with the numerical solution.