VM285
VM285
Determining the C* Integral in a Single-Edge Cracked Plate
Problem
Overview
| Reference: |
Kanninen, M.F., Popelar, C.H., Advanced Fracture Mechanics, Oxford University Press, p. 550, 1985 |
| Analysis Type(s): | Static Analysis (ANTYPE = 0) |
| Element Type(s): | 2D Structural Solid Element (PLANE182) |
| Input Listing: | vm285.dat |
|
VM285 requires a supplemental .cdb input file which is too long to include full input listings. This file must be downloaded and placed in your working directory for the test case to run properly. Additionally, the geometry and mesh should be regenerated. Download link: MAPDL Test Case Files for 2024 R2 vm285.cdb |
Test Case
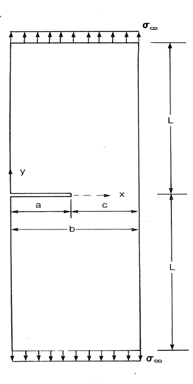
A rectangular plate with an edge crack is subjected to a tensile load σ∞ as shown in Figure 496: Two-Dimensional Fracture Problem Sketch. The load is applied instantly and held for 1000 hours. The C* integral is then determined using CINT and compared against the reference solution.
| Material Properties | Geometric Properties | Loading | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
Parameters for strain hardening creep
|
| σ∞ = 150 MPa |
Analysis Assumptions and Modeling Notes
The problem is solved using 2D structural solid elements (PLANE182) with plane strain element behavior. One half of the plate is modeled and symmetric boundary conditions are considered. The crack tip nodes and the number of paths surrounding the crack tip nodes are defined using CINT. The computation is completed in two steps. In the first step, the instal loading is applied on the top edge. In the second step, the load is held for 1000 hours. The C* integral is computed for the crack tip nodes by averaging the C* integral values from contour 2 to contour 9.
The reference value for the C* integral is determined using the following equation:
where:
| A = 5 x 10-12 |
| a = 2.5 |
| c = 17.5 |
| C1 = 17.5 |
| h1 = 8.57 (according to the reference material) |
| P = 3000 |
|
|
The reference value is therefore C* = 0.0319.