VM226
VM226
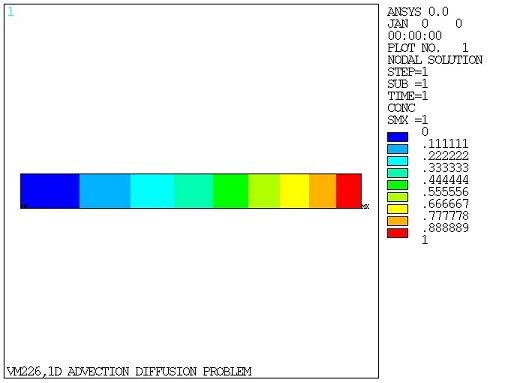
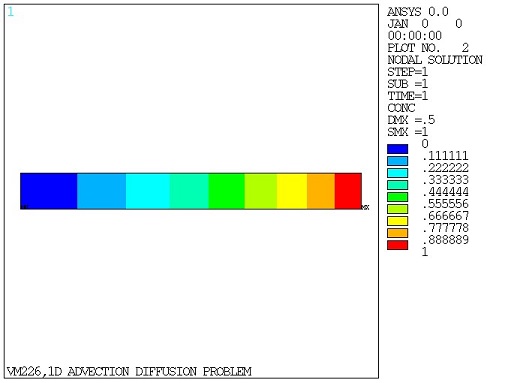
1D Advection Diffusion Problem
Overview
Test Case
Diffusion and mass transport effects due to the hydrostatic stress-migration are considered in a rectangular plane of length L and height H. Concentration constraints C1 and C2 are applied to the x = 0 and x = L ends of the plates, respectively. A steady-state analysis is performed to determine the distribution of concentration in the plate due to hydrostatic stress-migration.
| Material Properties | Geometric Properties | Loading | ||
|---|---|---|---|---|
|
Analysis Assumptions and Modeling Notes
Two approaches are used to solve this boundary value problem. The first solution uses a diffusion element type (PLANE238) and applies the hydrostatic-stress driving force V as a transport velocity body load (BF,VELO). The second approach uses a coupled structural-diffusion element type (PLANE223 with KEYOPT(1) = 100001). The UX DOF are constrained at location x = 0 m and the UY DOF are constrained at location y = 0 m and y = 0.1 m. An acceleration ACCELX is applied to the plate to create a stress-gradient in the X-direction. A Migration Model (TB,MIGR) is used to defined the volume of the migrating particle such that the transport velocity V is achieved.
Steady-state diffusion and structural-diffusion analysis is performed to determine the distribution of concentration in the plate. The obtained results are compared against the analytical solution at L/2.
For a 1D static case, the governing equation of diffusion is:
(226–1) |
This equation takes the form of a homogeneous second-order linear ordinary differential equation:
(226–2) |
The corresponding characteristic polynomial for this equation is:
(226–3) |
Considering the real roots,
(226–4) |
the generation solution of Equation 226–2 can be written as:
(226–5) |
Coefficients and
can be determined from the boundary conditions:
(226–6) |
Substituting Equation 226–6 into Equation 226–5, we obtain:
(226–7) |