VM-LSDYNA-SOLVE-037
VM-LSDYNA-SOLVE-037
Adiabatic Expansion of High Explosive Detonation Products Using Lagrangian
Approach
Overview
| Reference: | Lee, E.L., Hornig, H.C., & Kury, J.W. (1968). Adiabatic expansion of high explosive detonation products. Lawrence Radiation Laboratory, (1)1, 1-12. |
| Analysis Type(s): | S-ALE |
| Element Type(s): | 2D axisymmetric using Lagrangian approach |
| Input Files: | Link to Input Files Download Page |
Test Case
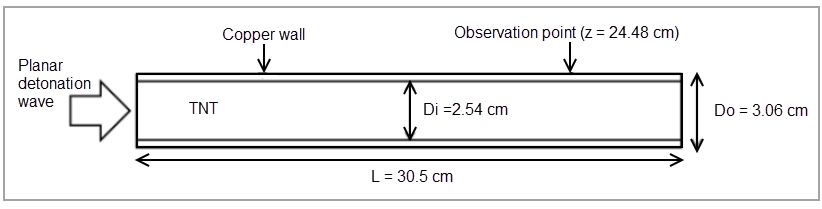
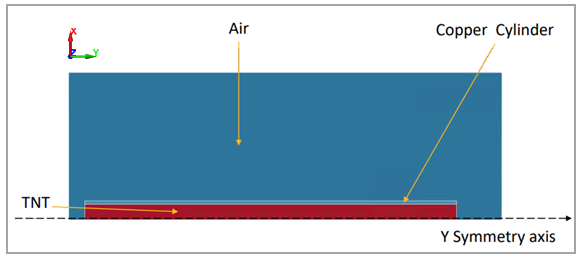
The setup involves a copper cylinder containing TNT explosive. Detonation begins at the base of the explosive using a planar detonation wave, which then travels along the axis of the cylinder. The radial expansion of the copper cylinder is measured at a specific point on its external surface (z = 24,48 cm). To measure the radial expansion, a 2D axisymmetric model with Eulerian formulation for the explosive and a Lagrangian modeling technique for the cylinder wall are used.
| Geometric Properties | Material Properties |
|---|---|
|
L = 30.5 cm Do = 3.06 cm Di = 2.54 cm |
Copper: ρ = 8.96 x 10-6 g/m3 G = 45.926 GPa ν = 0.35 |
|
TNT: ρ = 1.63 x 10-6 g/m3 D = 6930 m/s PCJ = 21 GPa | |
|
Air: ρ = 1 x 10-9 g/m3 |
where
| ρ is the material density |
| G is the shear stress |
| ν is the Poisson’s ratio |
| D is the detonation velocity |
| PCJ is the Chapman-Jouget pressure |
Analysis Assumptions and Modeling Notes
Since the model is axisymmetric, to simplify the process, a 2D axisymmetric structure has been considered. TNT explosive is detonated with a booster explosive generating a planar shockwave hitting the TNT, therefore, for simplification, detonation points are defined close enough to generate a planar detonation wave. For copper, the Gruneisen-Gamma EOS (Equation Of State) has been used. The values can be seen in Table 13.
Table 13: Gruneisen-Gamma EOS card
| *EOS_GRUNEISEN_(TITLE) (1) | |||||||
| EOSID | C | S1 | S2 | S3 | GAMMA0 | A | EO |
| 2 | 3940.0000 | 1.4890000 | 0.0 | 0.0 | 1.9700000 | 0.4700000 | 0.0 |
| V0 | UNUSED | LCID | |||||
| 1.0000000 | 0 | ||||||
For TNT, the Jones-Wilkins-Lee EOS is used. The values can be seen in Table 14.
Table 14: Jones-Wilkins-Lee EOS card
| *EOS_JWL_(TITLE) (1) | |||||||
| EOSID | A | B | R1 | R2 | OMEG | E0 | VO |
| 1 | 371.20999 | 3.7230000 | 4.1500001 | 0.9500000 | 0.3000000 | 7.0000000 | 0.0 |
For air, the Linear Polynomial EOS is used. The values can be seen in Table 15.
Table 15: Jones-Wilkins-Lee EOS card
| *EOS_LINEAR_POLYNOMIAL_(TITLE) (1) | |||||||
| EOSID | C0 | C1 | C2 | C3 | C4 | C5 | C6 |
| 3 | 0.0 | 0.0 | 0.0 | 0.0 | 0.4000000 | 0.4000000 | 0.0 |
| E0 | V0 | ||||||
| 2.500E–04 | 0.0 | ||||||
Copper material must be defined like a multi-material. See Table 16: Multi-material card.
Table 16: Multi-material card
| *ALE_STRUCTURED_MULTI-MATERIAL_GROUP_AXISM (4) | |||||||
| AMMGNM | MID | EOSID | UNUSED | UNUSED | UNUSED | UNUSED | PREF |
| COPPER | 3 | 2 | 0.0 | ||||
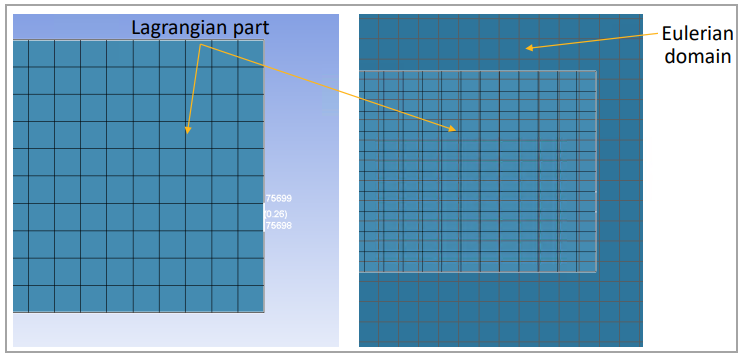
The mesh part overlaps the Eulerian domain and FSI is required to interact Eulerian domain with the Lagrangian part. The mesh model is shown in Figure 134 and the FSI card is shown in Table 17. The mesh size is 0.26 mm.
Table 17: FSI card
| *ALE_STRUCTURED_FSI (1) | |||||||
| COUPID | |||||||
| 0 | |||||||
| LSTRSID | ALESID | LSTRSTYP | ALESTYP | MCOUP | |||
| 2 | 1000 | 1 | 1 | -1 | |||
| START | END | PFAC | FRIC | FLIP | |||
| 0.0 | 1.000E+10 | 1.0 | 0.0 | 0 | |||
Results Comparison
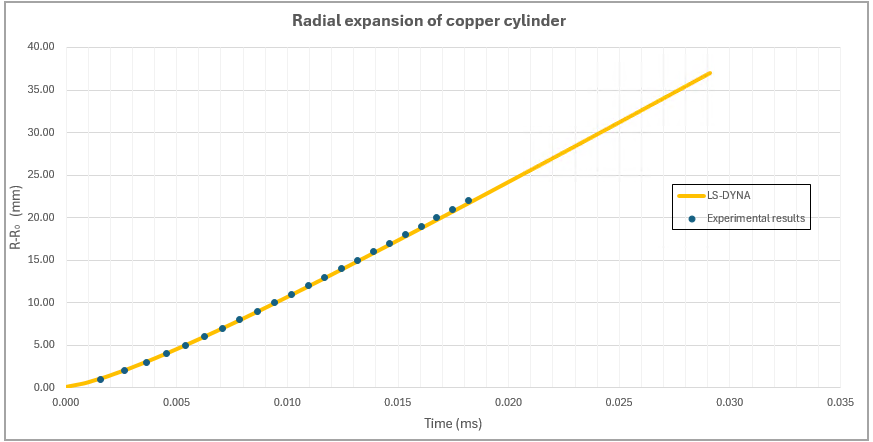
The results obtained by LS-DYNA are almost identical to the experimental results for R-R0—the radial expansion for the observation point at z = 24.48 cm from the moment expansion begins.
| Results | Target | LS-DYNASolver | Error (%) |
|---|---|---|---|
| R-R0 (mm) at observation point for time 16.05 ms | 19 | 18.78 | 1.17 |