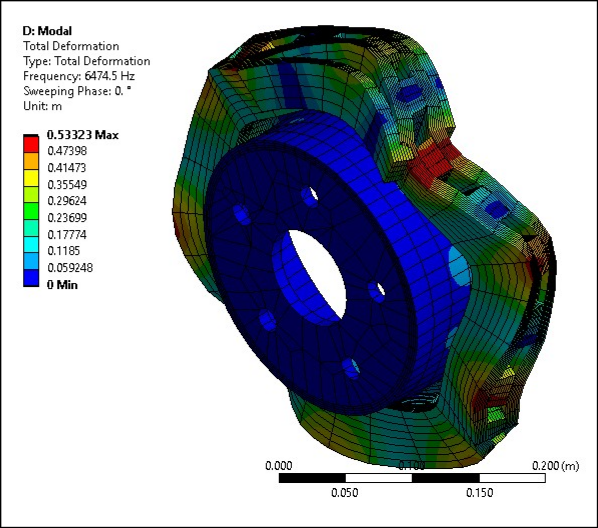
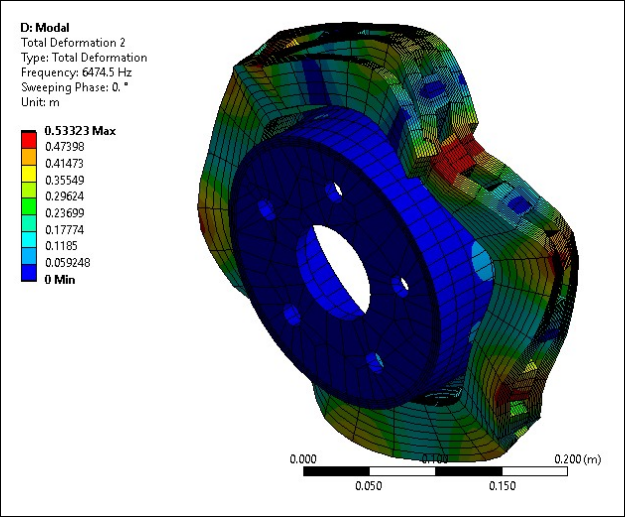
The unstable mode predictions for the brake disc-pad assembly using both methods were very close due to the relatively small prestress load. The linear non-prestressed modal solution predicted unstable modes at 6474 Hz, while the full nonlinear perturbed model predicted unstable modes at 6458 Hz.
The mode shape plots for the unstable modes suggest that the bending mode of the pads and disc have similar characteristics. These bending modes couple due to friction, and produce a squealing noise.
Table 1.1: Solution Output
| Linear non-prestressed modal | Full nonlinear perturbed modal | |||
|---|---|---|---|---|
| Mode | Imaginary | Real | Imaginary | Real |
| 1 | 775.91 | 0 | 775.32 | 0 |
| 2 | 863.54 | 0 | 863.18 | 0 |
| 3 | 1097.2 | 0 | 1096.7 | 0 |
| 4 | 1311.6 | 0 | 1309.6 | 0 |
| 5 | 1328.8 | 0 | 1326.1 | 0 |
| 6 | 1601 | 0 | 1600 | 0 |
| 7 | 1616.2 | 0 | 1615 | 0 |
| 8 | 1910.5 | 0 | 1910.5 | 0 |
| 9 | 2070.7 | 0 | 2069.7 | 0 |
| 10 | 2081.3 | 0 | 2080.2 | 0 |
| 11 | 2676.8 | 0 | 2671.2 | 0 |
| 12 | 2724.1 | 0 | 2718.1 | 0 |
| 13 | 3374 | 0 | 3371.9 | 0 |
| 14 | 4141.6 | 0 | 4140.9 | 0 |
| 15 | 4145.2 | 0 | 4144.8 | 0 |
| 16 | 4434.1 | 0 | 4422.4 | 0 |
| 17 | 4486.7 | 0 | 4477 | 0 |
| 18 | 4668.6 | 0 | 4665 | 0 |
| 19 | 4767.6 | 0 | 4765.2 | 0 |
| 20 | 5241.6 | 0 | 5240.9 | 0 |
| 21 | 6474.5 | -21.583 | 6458.2 | -21.788 |
| 22 | 6474.5 | 21.583 | 6458.2 | 21.788 |
| 23 | 6763.4 | 0 | 6762.8 | 0 |
| 24 | 6765.6 | 0 | 6765.2 | 0 |
| 25 | 6920.7 | 0 | 6916.8 | 0 |
| 26 | 6929.3 | 0 | 6929.1 | 0 |
| 27 | 7069.9 | 0 | 7057.1 | 0 |
| 28 | 7243.9 | 0 | 7239.8 | 0 |
| 29 | 8498.8 | 0 | 8478.5 | 0 |
| 30 | 8624.2 | 0 | 8592.2 | 0 |
It is important to determine the modal behavior of individual components (disc and pads) when predicting brake-squeal noise. A modal analysis performed on the free pad and free disc model gives insight into potential coupling modes. The natural frequency and mode shapes of brake pads and disc can also be used to define the type of squeal noise that may occur in a braking system. Bending modes of pads and disc are more significant than twisting modes because they eventually couple to produce squeal noise.
An examination of the results obtained from the modal analysis of a free disc and pad shows that the second bending mode of the pad and ninth bending mode of the disc can couple to create dynamic instability in the system. These pad and disc bending modes can couple to produce an intermediate lock, resulting in a squeal noise at a frequency close to 6470 Hz.