VM-EXD-MECH-004
VM-EXD-MECH-004
2-D Indirect Explosive Burn
Overview
| Reference: | Hand calculation based on shortest distance and detonation velocity |
| Analysis Type(s): | Explicit Dynamics 2-D |
| Elements: | Multi-Material Euler Quad |
| Boundary Conditions: | No |
| Structural Interactions: | No |
| Fluid-Structure Interactions: | No |
| Bonds: | No |
| Materials: | High Explosive (TNT) |
Test Case
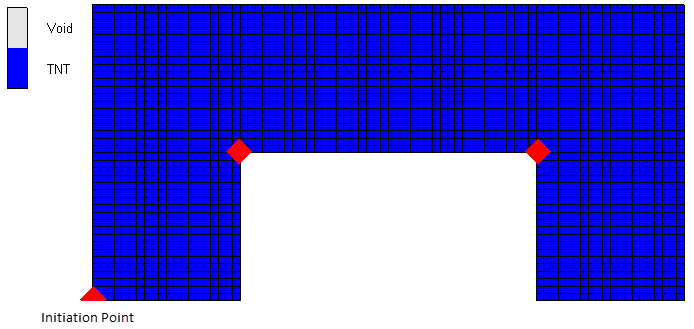
Detonation of an inverted, U-shaped block of high explosive (TNT) is initiated from a single point. The indirect-path method for detonation is used to ensure that detonation times are calculated correctly for all regions of the block. Additional detonation points are added to ensure maximum accuracy of the detonation-time calculations in regions that are not on a direct path from the initiation point.
Theoretical Solution
The detonation velocity for a high explosive is a known fixed quantity. Therefore, the detonation time at any point in the explosive is the time required for a detonation wave (traveling at the detonation velocity) to travel the shortest distance through the explosive region to that point.
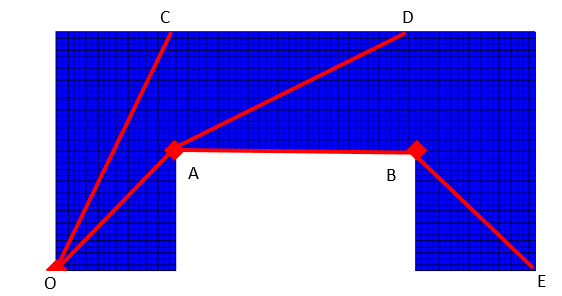
In this case, not all of the explosive is on a direct path from the single initiation point. For example, a straight line drawn from the initiation point to a point in the lower-right region of the explosive does not remain entirely within the explosive region but also spans the cut-out region:
To obtain meaningful and accurate results, therefore, it is necessary to use the indirect-path method for calculating detonation time.
Calculation Description
A single multi-material Euler part is created to model a region of high explosive (TNT). The external dimensions of the block are 40 mm x 20 mm. The dimensions of the cutout region are 20 mm x 10 mm.
A detonation point is defined at the lower-left corner of the block with an initiation time of zero. Two additional detonation points are defined at the corners of the cutout (as shown in Figure 231: Finite Element Model of U-Shaped Block of TNT and Detonation Points), for which initiation times are set to calculate automatically (from the first initiation). The indirect-path method for detonation is specified.
The material data for TNT is obtained from the Explicit Dynamics material library. The specified detonation velocity is 6930 m/s.
The calculation is run to t = 0.0069 ms.
Manual Results
The time for the detonation wave to travel from the origin to various points is calculated. Refer to the following figure:
Because points A and C have a direct line of sight to the detonation point O, the time of arrival of the detonation wave at A and C can be calculated using the detonation velocity and straight-line distance between O and A, or O and C, respectively.
Points B, D and E, however, do not have a direct line of sight to point O. The detonation time, therefore, is the time required for the wave to travel along the shortest path (consisting of multiple line segments).
Assume the following notation for any two given points (called I and J here) on the detonation block:
| tIJ – Time for the detonation wave to travel from I to J |
| LIJ – Straight-line distance between points I and J |
| ν – Detonation velocity for TNT (6930 m/s) |
The calculation for determining the time for the detonation wave to travel from origin point O to E, a point not within direct line of sight of O, is:
Calculation Results
Detonation times can be viewed during a calculation by contour plotting the variable ALPHA. The variable stores the detonation time of each element as a negative value.
The following plot of ALPHA at the start of the calculation shows that the detonation wave front follows the correct path through the explosive as it propagates around the cut-out region. The shortest distance the detonation front must travel (through explosive) to reach the lower-right corner of the block is 48.28 mm. At a detonation velocity of 6930 m/s, the detonation wave reaches this extremity at .0069 ms.
In Ansys Mechanical, the user-defined function DET_INIT_TIMESOLID shows the initial
detonation time of the nodes, useful for determining whether the detonation wave follows the
indirect-path method. Detonation occurs at 6.9382 x10-6 s.
| Results | Target | Ansys Mechanical | Error (%) |
|---|---|---|---|
| Detonation time for bottom right corner | 6.9382 x 10-6 | 6.9382 x 10-6 | 0 |