VMFL008
VMFL008
Flow Inside
a Rotating Cavity
Overview
| Reference | J.A. Michelsen. “Modeling of Laminar Incompressible Rotating Fluid Flow”. AFM 86-05., Ph.D. thesis. Department of Fluid Mechanics, Technical University of Denmark. 1986. | ||
| Solver | Ansys Fluent, Ansys CFX | ||
| Physics/Models | Laminar flow, Rotating reference frame | ||
| Input File |
| ||
| Project Files | Link to Project Files Download Page |
Test Case
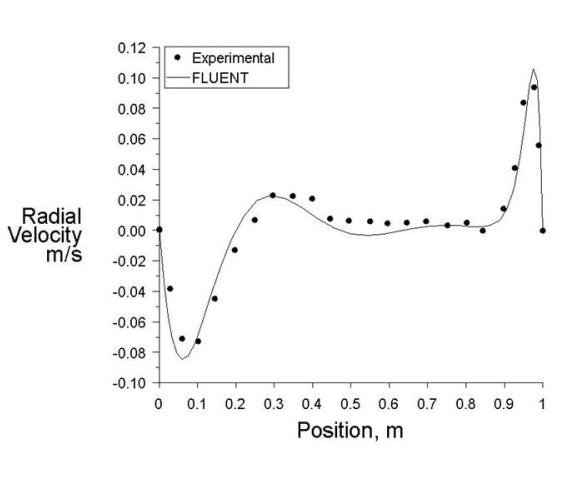
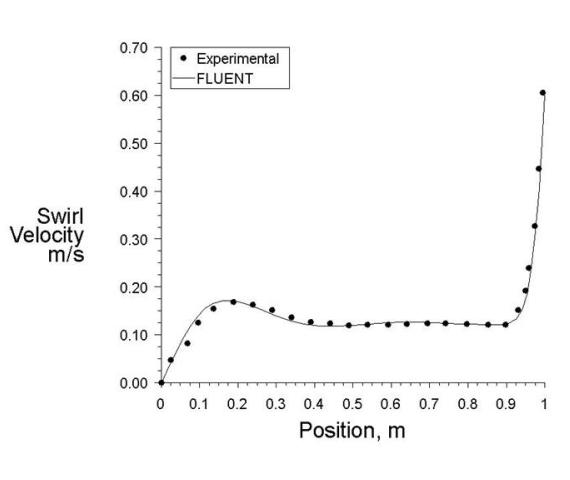
Flow in a cylindrical cavity enclosed with a lid that spins at Ω = 1.0 rad/s. The flow field is 2–D axisymmetric, so only the region bounded by the dashed lines in Figure 10: Flow Domain needs to be modeled. The Reynolds number of the flow based on the cavity radius R and the tip-speed of the disk is 1800.
| Material Properties | Geometry | Boundary Conditions |
|---|---|---|
|
Density = 1 kg/m3 Viscosity: 0.000556 kg/m-s |
Height of the cavity = 1 m Radius of cavity = 1m |
Speed of rotation of the moving wall = 1 rad/s Rotational velocity for cell zone = -1 rad/s |
Analysis Assumptions and Modeling Notes
The flow is laminar. The problem is solved using rotating reference frame.