VM17

VM17
Snap-Through Buckling of a Hinged Shell

Overview

Reference: C. C. Chang, "Periodically Restarted Quasi-Newton Updates in Constant Arc-Length Method", Computers and Structures, Vol. 41 No. 5, 1991, pp. 963-972.
Analysis Type(s): Static Analysis (ANTYPE = 0)
Element Type(s):
4-Node Finite Strain Shell Elements (SHELL181)
8-Node Finite Strain Shell Elements (SHELL281)
Input Listing: vm17.dat

Test Case

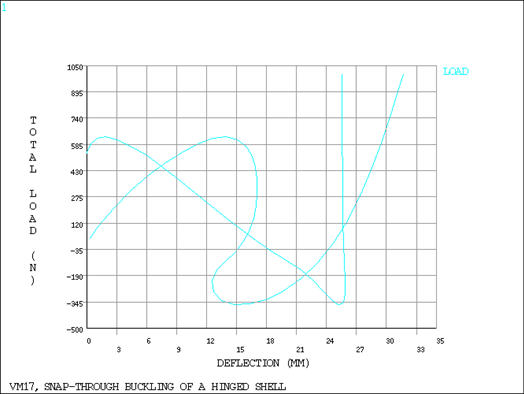
A hinged cylindrical shell is subjected to a vertical point load (P) at its center. Find the vertical displacement (UY) at points A and B for the load of 1000 N.

Figure 21: Hinged Shell Problem Sketch

Hinged Shell Problem Sketch

Material PropertiesGeometric PropertiesLoading
E = 3.10275 kN/mm2
υ = 0.3
R = 2540 mm
= 254 mm
h = 6.35 mm
Θ = 0.1 rad
P = 1000 N

Analysis Assumptions and Modeling Notes

Due to symmetry, only a quarter of the structure is analyzed. The structure exhibits the nonlinear post-buckling behavior under the applied load. Therefore, a large-deflection analysis is performed using the arc length solution technique. The results are observed in POST26.

Two analyses are performed, the first using low-order finite-strain shell elements (SHELL181), the second using high-order finite-strain shell elements (SHELL281).

Results Comparison

Target[1]Mechanical APDLRatio
SHELL181
UY @ A, mm-30.0-31.51.051
UY @ B, mm-26.0-26.91.035
SHELL281
UY @ A, mm-30.0-31.31.043
UY @ B, mm-26.0-26.51.021

  1. Target results are from graphical solution

Figure 22: Deflection and Total Load Plot

Deflection and Total Load Plot