VM110

VM110
Transient Temperature Distribution in a Slab

Overview

Reference:

F. Kreith, Principles of Heat Transfer, 2nd Printing, International Textbook Co., Scranton, PA, 1959, pg. 140, ex. 4-4.

Analysis Type(s):

Thermal Analysis (ANTYPE = 4)

Element Type(s):

3D Conduction Bar Elements (LINK33)

Convection Link Elements (LINK34)

Input Listing:

vm110.dat

Test Case

A concrete wall, originally at temperature T_o, is suddenly exposed on one side to a hot gas at temperature T_g. If the convection coefficient on the hot side is h and the other side is insulated, determine the temperature distribution in the slab after 14.5 hours, and the total heat Q transferred to the wall per square foot of surface area.

Figure 157: Slab Problem Sketch

Material Properties

Geometric Properties

k = 0.54 Btu/hr-ft-°F

ρ = 144 lb/ft³

c = 0.2 Btu/lb-°F

h = 5 Btu/hr-ft²-°F

= 1 ft

T_o = 100°F

T_g = 1600°F

Analysis Assumptions and Modeling Notes

The problem is first solved using LINK33 elements. A 1 ft² area is used for the convection and conduction elements. Node 12 is given an arbitrary location. Automatic time stepping is used. The initial integration time step (ITS) chosen (14.5/80 = 0.18125 hr) is larger than the minimum ITS recommended; ITS δ²/4 α, where δ is the conduction element length (0.1 ft) and a is the thermal diffusivity (k/ρc = 0.01875 ft²/hr). POST26 is used to obtain the total heat transferred to the wall.

Results Comparison

Time = 14.5 hr	Target[1]	Mechanical APDL	Ratio[1]
T, °F (node 1)	505.	507.	1.00
T, °F (node 3)	550.	549.	1.00
T, °F (node 5)	670.	675.	1.01
T, °F (node 7)	865.	874.	1.01
T, °F (node 9)	1135	1134.	1.00
T, °F (node 11)	1435.	1433.	1.00
Q[2], BTU/ft²	-20,736.	-20,662.	1.00

Based on graphical estimates
Q = 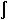q dt, from POST26