VM-DX-MECH-005
VM-DX-MECH-005
Optimization of Buckling Load Multiplier With CAD Parameters
and Young's Modulus
Overview
| Reference: | Timoshenko, Strength of Materials, Part 2 (Advanced theory and problems), pg. 167–168 |
| Analysis Type(s): | Goal Driven Optimization |
| Element Type(s): | 3-D Solid |
Test Case
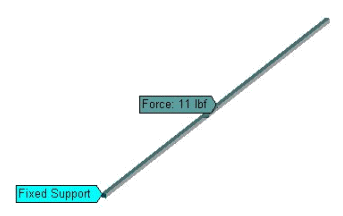
The cantilever bar of length 25 feet is loaded by uniformly distributed axial force p = 11 lbf on one of the vertical face of the bar in negative Z-direction. The bar has a cross-sectional area A is 0.0625 ft2.
Input Parameters: — Side of Square C/S, Length of Cantilever Bar and Young's Modulus
Response Parameters: — Load Multiplier of the First Buckling Mode
Optimization Method: — Genetic Algorithm
Sample Size: — 200
|
|
|
| Parameter | Type | Constraints | Desired Value | Importance |
|---|---|---|---|---|
| Cross-section side | Input | 0.225 ft.
| No Preference | N/A |
| Length | Input | 22.5 ft.
| No Preference | N/A |
| Young's Modulus | Input | 3.7594e9 psf
| No Preference | N/A |
| First buckling mode load multiplier | Output | N/A | Maximum Possible | N/A |
Analysis
Assuming that under the action of uniform axial load a slight lateral bucking occurs.
The expression for deflection is:
The critical load is given by,
where:
| q = force per unit length |
The first critical buckling load is:
The load multiplier is given by the ratio of critical load to
applied load
.
The first buckling multiplier is:
Combined objective function becomes:
Minimizing ϕ we get dimensions as:
| Cross-section side a = 0.275 ft. |
| Length l = 22.5 ft. |
| Young's Modulus E = 4.5948e9 psf |
| Buckling load multiplier = 3083.32 |