VM-LSDYNA-FLUID-003
VM-LSDYNA-FLUID-003
Steady 2D Laminar Flow around a Cylinder in a Channel
Overview
| Reference: | Schafer, M. & Turek, S. (1998). Benchmark computations of laminar flow around a cylinder. In Hirschel, E. (Ed.), Flow simulation with high-performance computers II. Notes on numerical fluid mechanics (NNFM) (547–566). Herzog-Heinrich-Weg 6, D-85604, Zorneding, Federal Republic of Germany. |
| Analysis Type(s): | Incompressible CFD |
| Input Files: | Link to Input Files Download Page |
Test Case
This test case reproduces a two-dimensional, steady, incompressible laminar flow around a cylinder inside a channel. The ICFD steady solver is used. The purpose of this test is to validate the drag and lift force coefficients and the pressure difference between the front and end points of the cylinder.
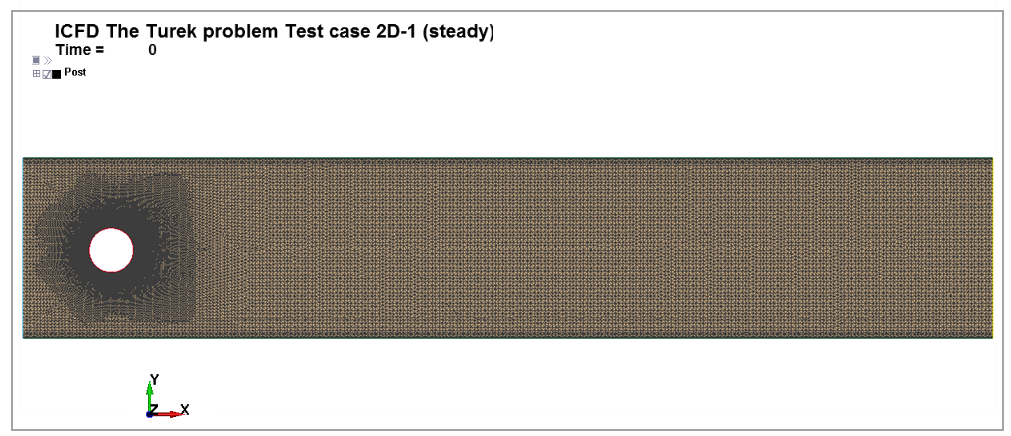
The domain consists of a rectangular box (the channel) with an inflow with prescribed velocity, an outflow with prescribed pressure and the two remaining sides of the boundary with non-slip conditions (channel's walls). The cylinder features a non-slip condition. The domain size and relevant quantities are shown in Figure 134. As you can see, the cylinder location is not perfectly symmetrical with respect to the channel height.
For this test case, all units of measure are consistent (length = m, time = s, mass = kg, force = N, pressure = Pa).
| Material Properties | Geometric Properties | Loading |
|---|---|---|
|
Fluid: • Fluid density ρ=1 • Inflow max velocity in channel’s axial direction Um = 0.3 • Flow dynamic viscosity μ = 10-3 |
Mesh size: • Fluid boundaries elements size: 0.008 • Cylinder elements size: 0.0005 Anisotropic elements added to cylinder • Boundary Layer: 5 • Anisotropic elements added to channel Boundary Layer: 3 • Geometry: Cylinder Diameter D = 0.1 Channel Height H = 0.41 |
Fluid: • Outflow pressure p = 0 |
Analysis Assumptions and Modeling Notes
The behavior of the flow is characterized by the Reynolds number:
(9) |
where ρ is the fluid's density, D is the characteristic length
of the problem (that is, the cylinder diameter), and μ is the dynamic viscosity of the
flow. is the flow mean velocity field at the channel's inlet.
(10) |
where U is the velocity field in the channel's axial direction which is imposed at the inlet (x = 0). The complete inflow velocity field relation is:
(11) |
where Um = 1.5 is the maximum inflow velocity in the channel's axial direction. This yields a Reynolds number Re = 20.
In this test, values of drag and lift coefficients (respectively, Cd and Cl) will be compared to the same values in the reference.
Nondimensional coefficients are evaluated using the following relation:
(12) |
where Fxx is the relative dimensional force on the cylinder surface. It's important to note that drag force is the resultant force on the cylinder in the asymptotic flow direction (that is, the x direction), lift force is the resultant force on the cylinder orthogonal to the asymptotic flow direction (the y direction), and both forces are the sum of the pressure and viscous forces.
As a further reference value, the pressure difference
is defined between the front and end points of the cylinder,
and
, respectively.
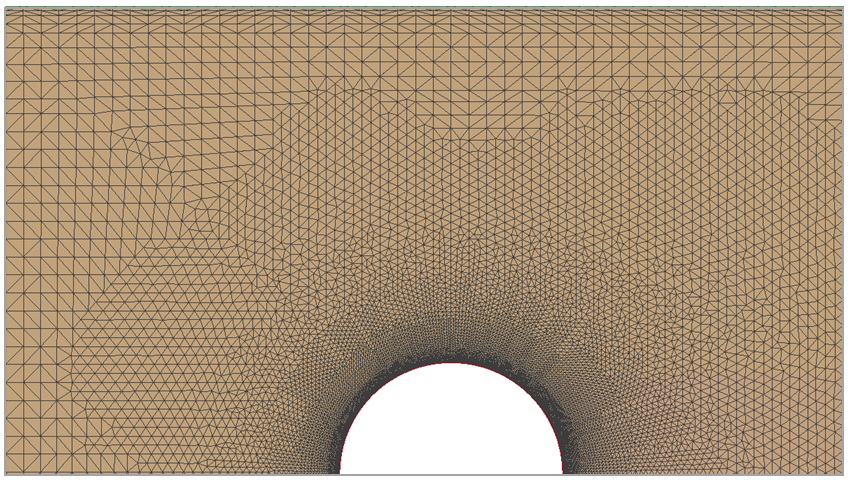
A picture of the domain's mesh is shown in Figure 135, while Figure 136 shows a detail of the mesh refinement around the cylinder and the channel's walls.
Results Comparison
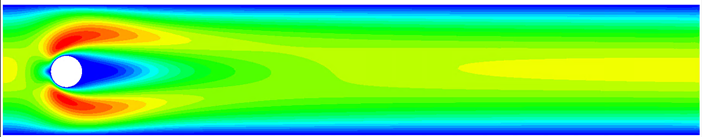
Figure 137 shows the flow velocity contour. Notice the symmetric, laminar, steady separation downstream of the cylinder. Also, note the boundary layer development along the channel's walls. Likewise, Figure 138 shows the pressure contour for the same case.
The table below compares the drag and lift coefficients (Cd and Cl) and the pressure difference ΔP between the present analysis and the reference. The reference proposes a comparison of the same values calculated with a variety of different numerical methods. The value shown in the target column, for each variable, is the average between its upper and lower bounds.
| Result | Target | LS-DYNA | Error (%) |
| Cd | 5.5800 | 5.5698 | 0,18% |
| Cl | 0.0107 | 0.0108 | 0.94% |
| ΔP | 0.1174 | 0.1174 | 0% |