VM24

VM24
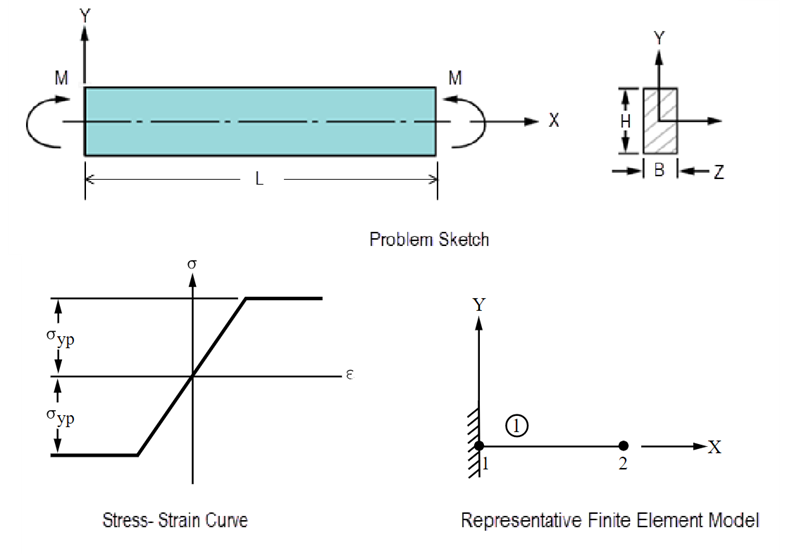
Plastic Hinge in a Rectangular Beam

Overview

Reference:	Timoshenko, S. (1956). Strength of Material, Part II: Elementary Theory and Problems (3rd Edition). New York, NY: D. Van Nostrand Co. (Article 64) 349.
Analysis Type(s):	Static, Plastic Analysis (ANTYPE = 0)
Element Type(s):	3D Plastic Beam Element (BEAM188)
Input Listing:	vm24.dat

Test Case

A rectangular beam is loaded in pure bending. For an elastic-perfectly-plastic stress-strain behavior, show that the beam remains elastic at M = M_yp = σ_ypbh²/6 and becomes completely plastic at M = M_ult = 1.5 M_yp.

Figure 30: Plastic Hinge Problem Sketch

Material Properties	Geometric Properties	Loading
E = 30 x 10⁶ psi ν = 0.3 σ_yp = 36000 psi	B = 1 in H = 2 in L = 10in I_z = b h³/12 = 0.6667 in⁴	M = 1.0 M_yp to 1.5 M_yp (M_yp = 24000 in-lb)

Material Properties

Geometric Properties

E = 30 x 10⁶ psi

ν = 0.3

σ_yp = 36000 psi

B = 1 in

H = 2 in

L = 10in

I_z = b h³/12 = 0.6667 in⁴

M = 1.0 M_yp to 1.5 M_yp

(M_yp = 24000 in-lb)

Analysis Assumptions and Modeling Notes

Bilinear kinematic hardening (BKIN) and 6 cells are used through the thickness.

An arbitrary beam length is chosen. Because of symmetry, only half of the structure is modeled (since length is arbitrary, this means only that boundary conditions are changed). The load is applied in four increments using a do-loop, and convergence status is determined from the axial plastic strain for each load step in POST26.

Results Comparison (for both analyses)

M/M_yp	Target Rotation	Mechanical APDL	Ratio
1.0	0.01200	0.01200	1.000
1.1666	0.01469	0.01459	0.993
1.3333	0.02078	0.02027	0.975
1.5	∞	0.11850	NA