In this example a simple coil spring model is held together by contact constraints. The initial contact status is open; therefore, the problem is initially underconstrained. As the load or boundary condition is applied, the contact changes to a closed status which stops any rigid body motion. However, if during any iteration in the nonlinear solve process the contact force becomes large enough to push the parts apart, the contact status will again open up and cause rigid body motion to occur.
The model is solved using five stabilization methods, and a comparison of results is provided. All methods give almost identical results. The quasi-static formulation is the most effective for this particular problem as it takes fewer iterations and does not require adjustment of solution parameters.
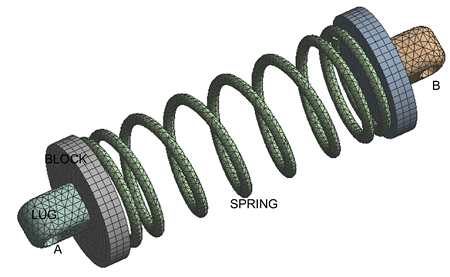
As shown in Figure 61.1: 3D Model of a Coil Spring System, the coil spring consists of lugs, blocks, and a spring held together only by contact constraints. The initially relaxed helical spring lies between the two blocks. The inner surface of the hole in lug B is fixed, and the inner surface of lug A is pushed 6 mm toward B and slowly released.
The connections between the spring and the blocks are modeled as asymmetric frictional contact pairs. The connections between the blocks and the lugs are modeled as always-bonded contact pairs.
All contact elements between the spring and the blocks may be in an open state during a solution step, which leads to temporary rigid body modes and the possibility of unreasonably large motion of the spring during the static solution.