The simplified viscoelastic model involves three material functions and a
parameter: the shear viscosity , the normal viscosity
, the relaxation time
, and a weighting coefficient
. Considering the empirical construction of the simplified
viscoelastic model, it is probably more relevant to inspect the behavior from the
point of view of extrusion flow, in particular from the point of extrudate swelling.
As will be seen, the various ingredients may have opposite effects, and enter in
competition with each other.
As is known, shear thinning decreases the extrudate swelling. However, the other
ingredients will usually enhance the swelling. Consider that the normal and shear
viscosities are the same function of their respective dependence variable; the
weighting coefficient and the relaxation time are to be considered. Actually,
selecting a normal viscosity independently with respect to the shear viscosity will
mainly make the analysis more complex without significantly affecting the general
conclusions. The weighting coefficient adjusts the intensity of the first normal stress difference, and
will essentially enhance the extrudate swelling. This is shown in Figure 6.71: Example of axisymmetric extrusion simulation for the simplified viscoelastic
fluid model through a cylindrical tube with a unit radius., which
plots the curve of swelling versus the flow rate for various simplified viscoelastic
fluid models. The continuous lines show the swelling for various values of the
weighting coefficient. Note that the amount of swelling can significantly be
affected. In Figure 6.72: Example of axisymmetric extrusion simulation for the simplified viscoelastic
fluid model through a cylindrical tube with a unit radius., note the development of the free surface versus the axial
distance, for various values of
. Note that the development versus the position is not
significantly affected be a specific choice of
. The relaxation time
appears in the transport equation for the viscoelastic variable;
consequently it will at first affect the development of the swelling along the flow
direction. This is visible in Figure 6.72: Example of axisymmetric extrusion simulation for the simplified viscoelastic
fluid model through a cylindrical tube with a unit radius.,
where the dashed lines indicate the development of swelling versus the axial
distance: the development distance increases with the relaxation time, while
actually the amount of swelling is less affected by the relaxation time. As can be
seen in Figure 6.71: Example of axisymmetric extrusion simulation for the simplified viscoelastic
fluid model through a cylindrical tube with a unit radius., the overall swelling is less affected by the value of the relaxation time.
Figure 6.71: Example of axisymmetric extrusion simulation for the simplified viscoelastic fluid model through a cylindrical tube with a unit radius.
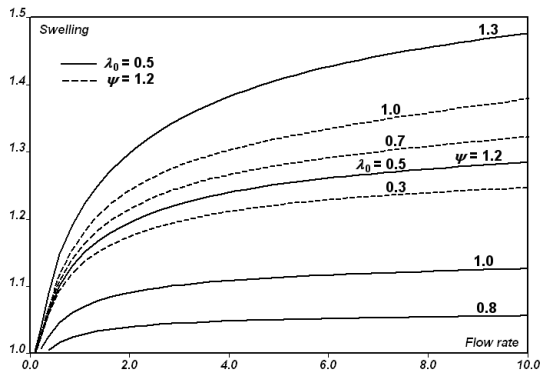
Swelling of an extrudate versus the flow rate, for various values of
(continuous lines) at
=0.5 and for various values of
(dashed lines) at
. Note that these curves are not obtained from Ansys Polymat; they
result from Ansys Polyflow Classic calculations.
Figure 6.72: Example of axisymmetric extrusion simulation for the simplified viscoelastic fluid model through a cylindrical tube with a unit radius.
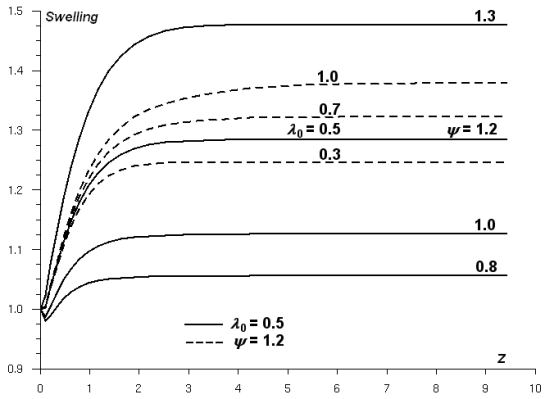
Development of an axisymmetric extrudate versus the axial distance at a flow rate
of 10 (see also Figure 6.71: Example of axisymmetric extrusion simulation for the simplified viscoelastic
fluid model through a cylindrical tube with a unit radius.), for various values of (continuous lines) at
=0.5 and for various values of
(dashed lines) at
=1.2. Note that these curves are not obtained from Ansys Polymat; they
result from Ansys Polyflow Classic calculations.


