VM-LS-DYNA-SOLVE-002
VM-LS-DYNA-SOLVE-002
Steady State Thermal Analysis of 3D Hollow Spheres with Heat Flux Boundary
Condition
Overview
| Reference: | Kreith, F. (1959). Principles of Heat Transfer (2nd ed.). International Textbook Co. |
| Analysis Type(s): | Steady State Thermal |
| Element Type(s): | Solid |
| Input Files: | Link to Input Files Download Page |
Test Case
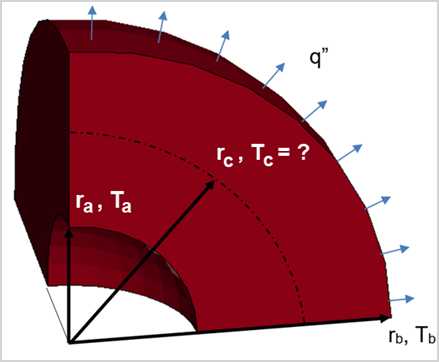
A hollow sphere has an inner surface temperature Ta of 100°C at ra. The material of the hollow spheres is isotropic. A constant heat flux boundary condition of 11.6091 W/m2 is applied on the outer surface rb. Compute the steady state temperature at rc = 3.51419 m.
| Material Properties | Geometric Properties | Loading |
|---|---|---|
| k = 1 W/(m°C) | ra = 2 m | Ta = 100°C |
| – | rb = 5.02839 m | q" = 11.6091 W/m2 |
Analysis Assumptions and Modeling Notes
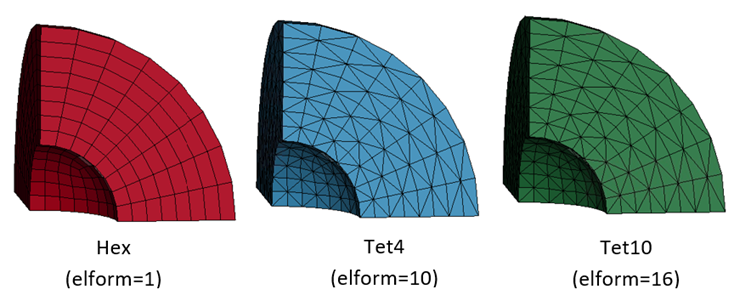
LS-DYNA Thermal Solver 11 is used. Three hollow spheres in this example are modeled using ELFORM = 1, 4, and 16. The temperature and flux boundary conditions are applied using the keywords *BOUNDARY_TEMPERATURE and *BOUNDARY_FLUX respectively.
The modeling of the sphere is accomplished using one-eighth symmetry. The heat flow is limited to be only in the radial direction.
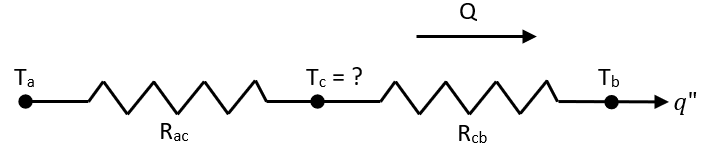
The rate of heat dissipation can be expressed as:
At steady state, the rate of heat dissipation Q:
Where the thermal resistance Rac can be expressed:
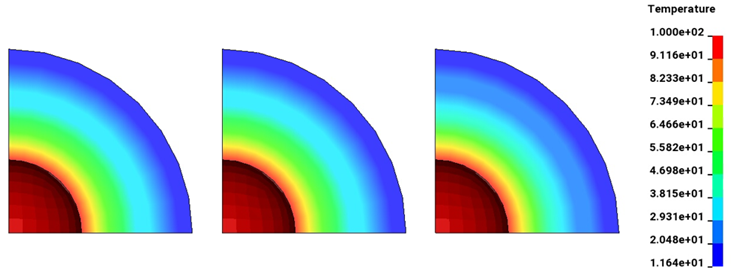
From the above equations, the analytical solution of Tc is obtained as 36.7617oC.