VM235

VM235
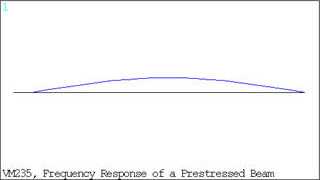
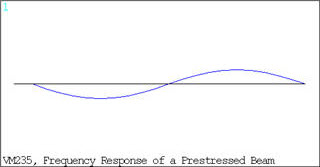
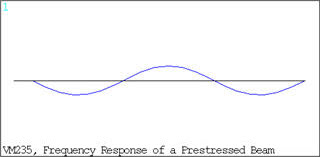
Frequency Response of a Prestressed Beam

Overview

Reference:

R. D. Blevins, Formulas for Natural Frequency and Mode Shape, Van Nostrand Reinhold Co., pg. 144, equation 8-20.

Analysis Type(s):

Modal (ANTYPE=2)

Element Type(s):

2D Elastic Beam Elements (BEAM188)

Electromechanical Transducer Elements (TRANS126 )

Input Listing:

vm235.dat

Test Case

A beam is in series with an electromechanical transducer. With a voltage applied to the beam, determine the prestressed natural frequencies of the beam.

Figure 393: Element Plot

Material Properties

Geometric Properties

Ex = 169E3 μN/μm²

DENS = 2332E-18 kg/μm³

Beam Stiff = 112666.667 μN/μm

Gap Stiff = 20.022 μN/μm

L = 150μm

W = 4 μm

H = 2 μm

Initial Gap = 1 μm

Gap = 0.999 μm

Volt = 150.162V

Analysis Assumptions and Modeling Notes

The beam is created using BEAM188 elements which are used in series with one TRANS126 element. The node that connects the transducer and beam is free to move in the X-direction, while the end node of the beam and the ground node of the transducer are constrained in both the X and Y directions. It should also be noted that the transducer gap stiffness is much less than the beam stiffness and will not have any effect on the frequency solution.

Figure 394: Mode Shape 1

Figure 395: Mode Shape 2

Figure 396: Mode Shape 3

Results Comparison

	Target	Mechanical APDL	Ratio
Frequency (mode 1)	351699.332	351609.996	1.000
Frequency (mode 2)	1381162.303	1379724.174	1.001
Frequency (mode 3)	3096816.034	3089542.986	1.002