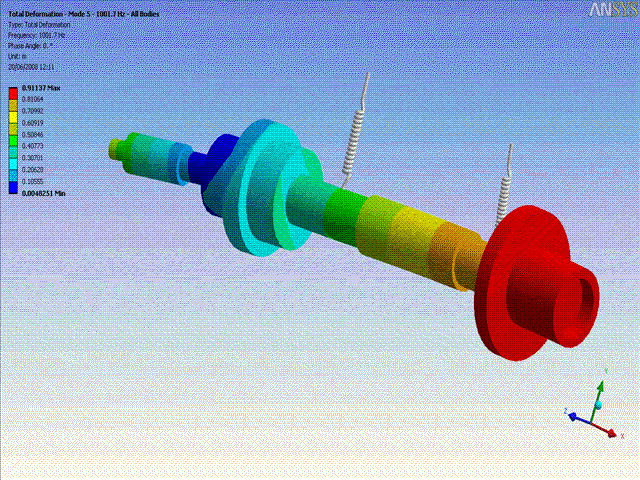
Rotordynamics is the study of vibrational behavior in axially symmetric rotating structures. Devices such as engines, motors, disk drives and turbines all develop characteristic inertia effects that can be analyzed to improve the design and decrease the possibility of failure. At higher rotational speeds, such as in a gas turbine engine, the inertia effects of the rotating parts must be consistently represented in order to accurately predict the rotor behavior.

An important part of the inertia effects is the gyroscopic moment introduced by the precession motion of the vibrating rotor as it spins. As spin velocity increases, the gyroscopic moment acting on the rotor becomes critically significant. Not accounting for these effects at the design level can lead to bearing and/or support structure damage. Accounting for bearing stiffness and support structure flexibility, and then understanding the resulting damping behavior is an important factor in enhancing the stability of a vibrating rotor.

The modeling features for gyroscopic effects and bearing support flexibility are described in this guide. By integrating these characteristic rotordynamic features into the standard FEA modal, harmonic, and transient analysis procedures, you can analyze and determine the design integrity of rotating equipment.

There are also specialized postprocessing features you can use to analyze specific behavior, and to process your simulation results to determine critical parameters. Orbit plots visualize the rotor's forward and backward whirl in a manner that allows you to easily determine the critical factors and the areas of concern. With the Campbell plots, you can determine critical speeds and system stability. These techniques, along with a number of other modeling and results analysis techniques are also covered in this guide.

Figure 1.1: Rotor Bearing System